
vol 8:
On money
Site map
Directory
Search this site
Home
1: About
2: Synopsis
3: Development
Next:
Previous: History: toc
This site is part of the natural religion project The natural religion project A new theology A commentary on the Summa The theology company
An essay on the divinity of money
Submitted to the Banking Law Association in competition for prizes to be awarded for the best papers on important issues of current banking law practice of relevance to Australia and/or New Zealand, may 1992
In questions of science the authority of a thousand is not worth the humble reasoning of a single individual. [Galileo Galilei (1632), quoted in Misner, C W et al, Gravitation, W H Freeman, San Francisco 1973 p 38.]
Contents
I A FRAMEWORK OF IDEAS
Theology
Science and Method
An old model of god
Cantor
Abstraction
The Limits to Mathematics
Revelation
Metamathematics
Completeness and Computability
Metaphysics
The Hilbert Oscillator
Knowledge
Parmenides
Physics
Cosmological Principle
Testing
How Universal is the Universe?
Locality and Uncertainty
Relativity
Quantum Mechanics
Computation
Conservation of Energy
The Principle of Least ActionII BANKING LAW
Law
The Rule of Law
Power
Money, good and work
Banking
Tax
Consumer Sovereignty
Discussion
Conclusion
Summary
1 Western theological tradition conceives of god as a personal being other than the universe who creates and controls our lives. Our political system still (rather tenuously) recognises god as a source of law through royal assent and the use of oaths. Since god is believed to be other than the universe, it cannot be observed and so cannot be the subject of science as we now understand it. Our information about god comes from ancient texts revealed to chosen prophets and interpreted by churches with equally ancient roots.
2 The rise of science questioned revelation and the churches as sources of truth, but they have remained in existence because science still lacks the power to ask or answer the fundamental questions of life and death that concern theology.
3 Here I outline a new scientific theology whose model of god derives not from ancient text but from the mathematical theory of text and communication itself. I propose that this model describes the universe of our experience, which is therefore fittingly called god.
4 I then interpret this model using elements of current physical theory. These ideas are then applied to money.
5 The movement of money is an abstract representation of the the activity of society as a whole, just as the flow of momentum in space-time is an abstract representation of the physical universe. My hypothesis is that proper understanding and political control of public cashflows is necessary and sufficient to obtain peaceful civilisation. (Back)
Introduction
6 This essay is a broad survey of ideas I hope to develop and publish one day in a more formal idiom.
7 The global failure of authoritarian socialist systems in competition with capitalism suggests that liberal democracy and the market are components of the ideal economic system, whatever that may be.
8 On the other hand currently acceptable political distortions to the market system show that the market alone is not considered sufficient to deliver peace and economic wellbeing to everybody. Should we distort the market, or is there another way of achieving economic stability, growth and distributive justice?
9 This essay is an attempt to re-examine the theoretical foundations of civilisation to see if it is possible to develop a more powerful model of peace and economic welfare. Civilisation teaches us how to live with people we do not know. (Back)
I A FRAMEWORK OF IDEAS
Theology
10 My starting point is theological. Briefly, theology is about god. God is the source of law. Law is the foundation of civilisation.
11 Let us say that god is the invisible or mysterious explanation of the existence and activity of the universe. Traditional arguments for the existence of god depend upon the premise that the observable universe is not self explanatory. Thus Aristotle, in his Metaphysics, was led to his first unmoved mover because he insisted that nothing could move itself, yet the world moves. (Aristotle XII: vi-vii)
12 Atheists say god is unnecessary because what we see is self explanatory. Yet we find that scientific explanations are possible and very valuable although not themselves observable. Our most powerful theories, quantum mechanics and general relativity, explain the flow of events using mathematical functions which are manipulated according to certain mathematical and physical rules.
13 We use these functions to model the world because they provide order among myriad data, predict the outcome of particular experiments and have much intrinsic beauty and harmony. We feel that they correspond to something real that controls the course of events and the outcome of experiments. On the other hand, scientific discipline requires that we assert no more than we can measure, and that we remain open to new models which may replace the familiar formalism with something else.
14 The existence of mysterious (non-observable) explanation, and the failure of ancient texts to give a consistent explanation of the mystery of our existence together suggest that there is room for a new theology. The real possibility of a scientific theology is here explored by producing a candidate model of god (MoG) for evaluation. (Back)
Science and method
15 Misner, Thorne and Wheeler claim that in physics
that view is out of date which used to say, "Define your terms before you proceed." All the laws and theories of physics ... have this deep and subtle character, that they both define the concepts they use ... and make statements about these concepts. Contrariwise, the absence of some body of theory, law, and principle deprives one of the means properly to define or even use concepts. Any forward step in human knowledge is truly creative in this sense: that theory, concept, law, and method of measurement - forever inseparable - are born into the world in union. (Misner, 71)
16 I feel that this statement applies to all of science, including theology. Each new birth has a past and arises out of problems in the old theory. A history of these problems is a history of science. Each is expressed and solved in the language of the day, but adds new meaning to old words. As modern physics has reinterpreted such ancient terms as physics, action, energy, momentum, space and time, a new theology would add new meaning to theology, god, creation, revelation, grace and the thousands of other words in the theological lexicon.
17 If we judge by output, the scientific enterprise is devoted to producing scientific literature. Science translates the language of nature into text readable by (at least some) humans.
18 Science is not the only human activity that reduces experience to symbolic form. The courts produce textual representations of events, and less formal producers of text are journalism, all the arts and the ceaseless everyday business of communication through all the forms of language and symbolism known to humanity.
19 This suggests that formal arrangements of symbols can successfully represent or model nature. We have a promising model when the observable consequences of manipulating the text according to its formal rules are found to match the observable outputs of natural process. If nature can be modelled by a formal system, it seems reasonable to assume that nature itself is in some way a formal system.
20 If this is so, it is plausible that mathematical results that apply in formal systems qua formal systems will apply also in nature. We should therefore expect to find in nature properties and limitations akin to the limitations to formal systems discovered by Goedel, Turing and others.
21 The scientific process is asymmetrical. A good theory must be open to test by observation, that is falsifiable. (Popper, 33) While a model may be rejected on the grounds of one false prediction, its success does not exclude the possible existence of another model with the same observable input and output. This situation arises from our ignorance of the complexity of nature.
22 We need not be so ignorant of formal systems of our own construction. If we can arrange that there are only two possibilities, say by dividing our whole universe of discourse into p and not-p, we can use the inconsistency of one possibility to prove the truth of the other. This approach is the basis of non-constructive or indirect proof in mathematics. This form of proof, often called reductio ad absurdum, was known to Aristotle and was used well before his time to show that the square root of two is irrational. (Kramer, 27)
23 A similar form of argument is essential to theology where it is known as the negative way or via negativa. As Aquinas puts it:
... since ... we cannot know what god is, but what it is not, we cannot talk about how god is but rather how it is not. ... It is possible to show how god is not by removing from it things which are not proper such as composition, motion and other similar attributes. (Summa Theologiae I q I a 2) (Aquinas)
24 Falsifiability, non-constructive proof and the via negativa are a common thread which suggests a route to a scientific mathematical theology. (Back)
An old model of god
25 The details of the Catholic god derive as much from ancient Greek thought as from the Bible. The Greeks made a deep study of the relationship between invariance and motion which is modelled by the mathematical relationship between discrete and continuous.
26 The study of continuity was a mature science in Zeno's day, 2400 years ago. Greek science was not so compartmentalised as things are today, and the study of nature (physics) and the study of knowledge (metaphysics) were considered complementary.
27 The philosophers sought certain knowledge, that is knowledge not subject to revision or change. To remain true, unchanging knowledge must derive from unchanging reality. So if certain knowledge exists the foundations of reality must be eternal, outside time and change.
28 The Greek scientific community divided at this point. Some decided that the whole of reality is eternal and that the motion we perceive is an inferior form of existence or an illusion. Others sought to understand how the world of physics could be both still and moving and the world of metaphysics both certain and uncertain.
29 The first group is called rationalist. A rationalist prefers rational argument to experience if the two appear to contradict. Zeno was a student of Parmenides. Parmenides is believed to have held that the multiplicity of existing things, their changing forms and motion, are but an appearance of a single eternal reality ('Being'). Zeno's paradoxes clearly exposed some of the problems involved in a rational understanding of motion and continuity.
30 The other group is represented here by Aristotle. Aristotle took up the study of motion in his Physics and established, by definition, a relationship between continuity and infinity what is infinitely divisible is continuous (Physics Book III:1 (Aristotle)). He then went on to develop his doctrine of potency and act (Metaphysics, Book IX:1 (Aristotle)) to explain motion, but failed to produce a satisfactory treatment of continuity and infinity.
31 This failure meant that rationalism, in the form of Platonism, became the dominant input into the theoretical development of Catholicism. Parmenides' eternal being became the foundation for the Catholic model of god and the visible universe took on a 'second best' status.
32 The Catholic god is completely other than the universe and not therefore observable. Instead of observation, the Catholic theologian must rely for data on official interpretations of the Bible and other traditions provided by the Catholic Church (Code of Canon Law Canon 749) A scientific theology is thus not possible in the Catholic milieu and one finds that experience is subservient to dogma in many other traditional religions. (Back)
Cantor
33 The modern inquiry into continuity began with the invention of calculus and culminated in the nineteenth century in the work of Georg Cantor. Cantor's study of the continuum led him to the theory of sets and transfinite symbols. (Cantor)
34 Cantor defined a set (Menge) to be any collection into a whole M of definite and separate objects m of our intuition or our thought (ibid, 85). He then defined two abstract representations of set, cardinal number and ordinal type, and explored their properties.
35 The cardinal number of M, card(M), is the general concept which, by means of our active faculty of thought, arises from the aggregate M when we make abstraction of the nature of its various elements m and the order in which they are given (86). Sets with finite cardinal numbers are called finite sets; all others are called transfinite sets and their cardinal numbers transfinite cardinal numbers represented by aleph, the first letter of the Hebrew alphabet.
36 The ordinal type of a set M, ord(M), abstracts from the nature of its elements m but retains their ordering. Cantor believes that
The concept of 'ordinal type' ... embraces, in conjunction with the concept of 'cardinal number' ... , everything capable of being numbered that is thinkable, and in this sense cannot be further generalised (117) .
37 The smallest transfinite cardinal aleph(0) is the least upper bound of the finite cardinal numbers 0, 1, 2, 3 ... . Using the theory of ordinal types, Cantor showed that given any transfinite cardinal number aleph(m) there exists a set of higher cardinal numberaleph(m+1) Cantor's theorem (CT) establishes an ordered and unending abstract hierarchy of cardinal and ordinal numbers which is called the Cantor Universe (CU).
38 I propose to use the CU for my MoG. The rationale for this is that both god and the CU are defined non-constructively. CT tells us that given the countably infinite set of natural numbers it would be inconsistent for the other transfinite numbers not to exist. Aquinas, the Catholic Church and millennia of mystical tradition tell us that we can only talk about god by the via negativa, removing inconsistencies.
39 My purpose in this article is theological, not mathematical. Like a physicist, I treat mathematics as a store of possible models to be exploited without too much respect for mathematical finesse. All my logical steps must be tested, and the model itself must be interpreted in a way which yields testable physical predictions. (Back)
Abstraction
40 Following Cantor, we may distinguish four levels of abstraction. The zeroth is concrete reality, not abstract at all but included for completeness. The first is mental or imaginative, and corresponds to the mental images we have of concrete realities such as trees and stones. It is at this level that we imagine sets such as a set of trees or stones. The second and third are ordinal and cardinal numbers as defined.
41 Each level of abstraction is a model of those before it and a realisation of those after it. I propose the CU as a model of the universe, or equivalently, that the universe is a realisation of the CU. This hypothesis suggests that I may identify god and the universe, since the CU is my MoG.
42 A model abstracts from the detail of reality. If the model is good, this abstraction gives a true account of reality, and manipulations of the model reflect reality. If the CU is a good model of the universe, we should find that the laws of cardinal and ordinal numbers established by the theory of sets hold in reality. In fact we have never found any practical flaws in the working of arithmetic, which underpins the whole accounting function in commerce and all computations in science, engineering and all other disciplines.
43 When we talk about the properties of numbers, we also talk about properties of the real world. This fact has fascinated scientists (and accountants and entrepreneurs) for a long time. Einstein said The most incomprehensible thing about the world is that it is comprehensible (Schilpp 112). The proposition under examination here, that we can conceive of the observable universe as an implementation of all possible consistent symbolic structure, provides us with some insight into the comprehensibility of the universe. (Back)
The Limits to Mathematics
44 Although Cantor's theory is quite simple, it soon began to throw up paradoxes that forced a careful re-examination of the foundations of mathematics. The result of this re-examination, which continues today, is that there are limits to mathematical certainty.
45 A most productive attack on the foundation problem was led by David Hilbert. Hilbert took the formalist point of view, which regards mathematics as a purely formal game played with marks on paper. For a formalist, mathematics is text about text, and so is bound by its own discoveries.
46 The only rule is to avoid inconsistency. The assumption behind this approach was that the paradoxes of set theory lay concealed in the semi-natural language used by the mathematicians of the day. By eliminating natural language altogether, Hilbert hoped to eliminate the hidden paradoxes.
47 By 1928 Hilbert was able to encapsulate his thoughts on the nature of mathematics in three questions:
q1 Is mathematics consistent?
q2 Is mathematics complete?
q3 Is mathematics computable?
48 He believed that the answer to all three questions would be yes, proving that there were no limits to mathematics. He was to claim in 1930 that there is no such thing as an unsolvable problem (Hodges, 92).
49 Goedel and Turing destroyed this belief. Consistency in mathematics can only be bought at the expense of incompleteness and undecidability, just as consistency in quantum mechanics requires us to accept uncertainty. I feel that these results are related and that the exploration of this relationship may lead to a new understanding of motion and stillness and open the way for a new understanding of god. (Back)
Revelation
50 Revelation is the traditional foundation of theology. Catholicism understands revelation to be direct knowledge of god revealed to prophets and passed on by them to non-prophetic individuals (Abbott,111). God, although not normally observable, becomes observable to the prophet through a special process known as inspiration.
51 Here revelation will mean scientific knowledge, which includes the aggregate of a priori knowledge we call logic and mathematics. The essential content of a priori knowledge is the boundary of consistent formal systems defined by the aggregate of all proofs.
52 Like the mathematical models of physics, this knowledge does not come to us directly through our senses but through the mental processing of sense data. It is abstract knowledge which may be represented by marks on paper or any other medium.
53 This revelation is my MoG, the aggregate of all consistent formal structures. Such revelation is available to all rather than a few chosen ones. This understanding of revelation is not inconsistent with the ancient belief that the light of human intellect is participation in god (Aquinas, I:79:4). (Back)
Metamathematics
54 Practical mathematics lies in the realm of consistency. The study of the boundaries of mathematics as pursued by Hilbert and others is called metamathematics. Metamathematics tells us just how far we can go with formal systems without abandoning consistency. The establishment of consistency is called proof, so that it is for proof to tell us how far proof can go.
55 CT is the foundation of symbolic operations because it establishes the existence of the number system upon which all else is built. The numbers are an ordered set of discrete symbols. CT holds for consistent systems of symbols. We may think of it as a source of symbols, here called the Cantor source, CS. (Back)
Completeness and Computability
56 Goedel and Turing showed that some of the apparently pathological behaviour which Hilbert attributed to natural language is essential to consistent formal systems. Mathematics is complete if every mathematical statement that obeys the formal rules can be either proved or disproved. Mathematics is computable if there exists a definite mechanical process, like the execution of a computer program, which can decide whether a given proof is valid or not. The proof of completeness is thus logically dependent on the proof of computability.
57 Turing proved that mathematics contains incomputable statements by devising a universal machine that could perform all possible logical operations and showing that there were proofs that this Turing Machine could not complete. Turing concentrated on computable numbers, since they allowed a simplified form of a proof which also applies to functions, predicates and, in fact, any formal symbolic system. Turing considered a number computable if its decimal could be written down by a machine.
58 Using the structure of the Turing machine as a mapping tool, Turing transformed the problem of computability into a question about the relationship between aleph(0), the cardinal number of the set of rational numbers and aleph(1), the cardinal number of the set of reals, using the diagonal argument pioneered by Cantor. I will approach the result more simply using algorithmic information theory.
59 Algorithmic information theory defines the algorithmic information content I(x) of x as the size of the smallest program to calculate x. (Chaitin, 55) It then invokes an idea similar to the law of requisite variety in cybernetics (Ashby, 202-216): if a theorem contains more information than a given set of axioms, then it is impossible for the theorem to be derived from the axioms.
60 In the case of the Turing Machine, this principle shows that a system whose complexity or information content is measured by aleph(1) cannot be computed by a system whose complexity is measured by aleph(0).
61 We can extend this argument to get a transfinite hierarchy of computability. This generalization of Turing's argument says that for n > m, a system whose complexity is measured by aleph(n) cannot be computed by a system whose complexity is measured by aleph(m).
62 Using the CU, complexity and computability, we can produce a rudimentary description of the universe. Insofar as the universe is consistent, the CS is on and emits symbols of ever increasing complexity. This looks like the 'big bang', starting simple and growing more complex. CT tells us that any formally consistent system must create greater and greater complexity.
63 Now assume that one system A may know another system B only insofar as B is computable using the resources of A. Assume further that insofar as the complexity of B is beyond the computing resources of A, we are justified in calling B mysterious relative to A . Since we know from CT that given any system X there must be a system of greater complexity Y, we are guaranteed the existence of mystery for any system. This, in outline, is my proof for the existence of god.
64 As I interpret it, CT requires that any infinite consistent formal system be a source of complexity, that is be creative. The ancients such as Aristotle could not see how the visible universe could create itself and therefore postulated god as the invisible creator. Now we can say that insofar as the universe can be modelled by a consistent formal system it must express CT and so create itself. We need not understand how this is so, but simply accept that it is inconsistent for a formal system to be otherwise. (Back)
Metaphysics
65 I take metaphysics to be primarily the study of knowledge. All men by nature desire to know (Aristotle, Metaphysics I:1). I am an individual component of the universe seeking to reflect its structure in myself and express my reflection in this text.
66 CT guarantees that a consistent formal system gives rise to the CU. We may conceive the CU as a static formal space existing in the world of mathematical symbols.
67 I now wish to take a further step toward reality by extending the model to account for the process of modelling as I experience it and apply the extended model to the universe as a whole.
68 I do this with a device I call the Hilbert Oscillator (HO) which operates between different levels of the transfinite hierarchy. The increase in complexity is driven by CT. The decrease in complexity, corresponding to abstraction, knowledge or modelling is driven by a theorem of mathematical communication theory known as the E theorem. (Khinchin, 54-58) (Back)
The Hilbert Oscillator
69 Figure 1 is a first version of a formal representation of the HO. It is based on Hilbert's questions is mathematics consistent? and is mathematics computable? The four states of the oscillator 0-3 simply represent the four possible combinations of yes-no answers to these two questions in their natural binary order.
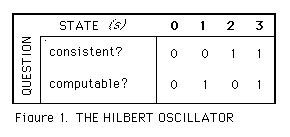
70 Let us attempt an intuitive correlation of these states with the search for understanding. We begin with a problem, a situation best represented by state 1. In this state one is certain that there is inconsistency - a previous belief has been successfully falsified.
71 The next step is to consciously and unconsciously permute the set of known facts or observations in an attempt to arrive at a consistent understanding. This corresponds to state 0, a state of darkness lacking both consistency and computability. Einstein gave us a classic expression of this and the next phase: The years of searching in the dark for a truth that one feels but cannot express, the intense desire and the alternations of confidence and misgiving, until one breaks through to clarity and understanding are known only to him who has himself experienced them (Pais, 257).
72 The breakthrough to clarity corresponds to the reestablishment of consistency and the ability to see order in an infinite number of observations from the new point of view. This corresponds to state 2. Finally the new insight can be reduced to writing and definite methods of computation, corresponding to state 3
73 Experience shows that this point, the answer to the original problem, gives rise to a large number of further problems. The volume of scientific work awaiting completion grows rather than diminishes with each discovery.
74 Figure 2 is an attempt to summarise this interpretation of the Hilbert cycle in a manner reminiscent of the temperature-entropy diagram used in thermodynamics.
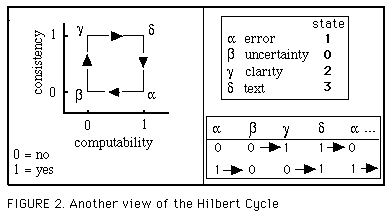
75 We now turn to establishing a more formal relationship between the Hilbert cycle and the Cantor Universe.
76 CT interpreted dynamically establishes the existence of the CF, which acts to increase the complexity of the universe. Since the source of the CF is consistency, it acts at every point in the universe. We may understand the CF as the foundation of the second law of thermodynamics which tells us that the entropy of the universe never decreases (and usually increases) in any process. (Back)
Knowledge
77 The force driving knowledge, the desire to know, is the inverse of the CF. Let us call this the E Force (EF) because it corresponds to the E-property. Knowledge is to some degree abstract and general. Knowledge is simpler than the thing known because we abstract from individual detail. As a consequence a particular item of knowledge applies to many individuals, and hence is general. So we have principles of accounting which apply to all enterprises because they abstract from the details of individual businesses.
78 In the light of the theory of evolution, we explain the natural desire to know by the competitive advantage that knowledge confers. An organism with knowledge of its environment is in a better position to predict future events and adjust its behaviour accordingly. The mathematical theory outlined here suggests that knowledge is not simply confined to living organisms, but is a fundamental component of all symbolic systems, and of the universe insofar as it is modelled by text.
79 The transfinite cardinal numbers aleph are a natural measure of complexity. Their exponential structure makes a nice mathematical fit with the logarithmic scale usually chosen to measure entropy and complexity (Khinchin, p 2).
80 A communication system is modelled as a source and a channel. Shannon founded information theory on a consideration of Markov sources. Khinchin extended the treatment to any ergodic source and any stationary channel with finite memory (Khinchin 30). Here I speculate on applying the theory to the CU. Space constrains me to speak only of sources.
81 Communication theory is an application of probability theory (Kolmogorov). The output of a source is regarded as a random process. We take no heed of any 'meaning' which may be encoded in the output. The probabilistic structure of this process constitutes the mathematical definition of the given source.
82 The first part of the definition of source S is a set A of symbols called the alphabet of S whose elements are called letters. The second part of the definition of S is a measure of the probability of emission of sequences of letters, mu.
83 From this probability structure we can compute a number H called the source entropy which is a measure of the average amount of information carrying capacity per letter of the source output. The source is said to be stationary if the probability regime of its output does not change with time.
84 The only property of a source which concerns is here is the E-property established by McMillans's Theorem (Khinchin, 54-58). The E-property divides the set C of n letter words in the output of a given source into two groups known as the high probability group and the low probability group.
85 With the exception of the case when all the letters of the alphabet are equiprobable we find that for large n the high probability group contains only a negligibly small share of possible n letter words from the source. Almost all words fall into the low probability group. On the other hand, most of the entropy of a source is carried by words from the high probability group.
86 This fits our experience. Day to day english speech uses only a few thousand different words out of the millions we find in big dictionaries. Some little words used very frequently carry most of the traffic. The E-property is not confined to natural language, however, but applies to all ergodic sources.
87 Mathematics is a timeless formalism. A correct proof establishes relationships between symbols which are eternally valid. A source, on the other hand, emits a sequence of letters in time. By the time you have spoken the last sound in a sentence the first has died away. It no longer exists, and so cannot be talked about in the eternal present of mathematics.
88 It has become customary in physics and mathematics to represent such time sequences by a mathematical space. In figure 4 below, for instance, the time axis is drawn just like the space axis, and we treat all instants of time as existing simultaneously just like all the points in space. In general terms, a source is ergodic if we can satisfactorily study its behaviour in time through arguments in space.
89 Now without argument or proof, let us assume that the E-property allows us to encode almost all the information in a set of complexity aleph(n) into a set of complexity aleph(n-1). This encoding (which would be performed by a computing machine) I use as a model of knowledge.
90 It fits our experience of knowledge. Knowledge reduces complexity by abstracting the salient features of a situation. These salient features correspond in our model to the high probability sequences. Knowledge is an imperfect representation of reality. There is some information (corresponding to the low probability sequences) lost by confining our attention to the high probability sequences.
91 The number of different low probability sequences which lie outside the realm of knowledge is much greater than the number of high probability sequences, although the low probability sequences are much rarer and more interesting. This corresponds to the common belief that the scope of the mystery in the universe is much greater than what can be known.
92 We are now in a position to draw a third picture of the HO.
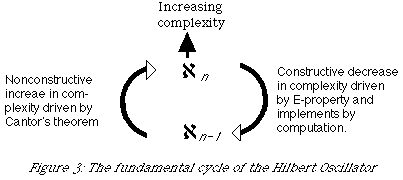
(Back)
Parmenides
93 Armed with this elementary model of the universe and a model of knowledge within it, I wish to look again at Parmenides' position. All we have from him are fragments of a long didactic poem. He begins with an allegory of his chariot journey through a gate leading from night to day, where he is welcomed by a goddess whose words form the rest of what we've got.
94 She tells him (Hammond,782):
Only three methods in philosophy ... are conceivable, viz. (to assume) that (the reality to be studied) necessarily is or that it necessarily is not or that it both is and is not, comes to be and perishes, changes and moves. The last two methods are excluded by the argument that only what is and cannot not be can be known. The goddess therefore proceeds to a summary deduction of the characteristics of what is; given that it necessarily is whatever it is, it must be ungenerated and imperishable, indivisible, self-identical, unique, motionless, determinate, perfect and in perfect equilibrium like a solid sphere. It follows that many things of which mortals speak as being and not being, etc (i.e. as having a contingent and relative being), exist only in name. (my emphasis)
95 I think we can now add a little to this story. From the principle that only what is and cannot not be can be known Parmenides deduces that reality must be motionless. If we demand that the universe be consistent, however, we find that motion too becomes necessary. The formal path this conclusion is through CT, which tells us that a given transfinite number must generate its successors and that generation is motion.
96 How do we reconcile motion and stillness? The formal answer, I believe, is that we cannot. We are here face to face with mystery. We have some evidence for this mystery in physics. Heisenberg's uncertainty principle tells us that there is a fundamental limit to the precision with which we can measure certain quantities. (Back)
Physics
97 Physics studies natural motion. It seeks the invariant structure of the changing universe which we call principles and laws. Because these laws are invariant they can be written down and remain through time, whereas the motions they describe cannot.
98 We know they are there because our lives are stable as well as changing. We look for them because we are curious, and because knowing them usually pays off handsomely. Knowing the law is like having the street directory of a big city - journeys from point to point become much quicker.
99 The whole of our present way of life depends upon the discovery of fossil fuels and heat engines, which were brought together by the eternal laws of thermodynamics. And where would we be without our quantum mechanical understanding of lasers and semiconductors? (Back)
Cosmological principle
100 This essay revolves around two cosmological principles which I will call the Cantor cosmological principle and the ergodic cosmological principle.
101 Cosmological principles are a scientific antidote to human self-centredness. (Silk, 2-6) When we first became conscious, we considered ourselves to be at the centre of the universe. Since then observational astronomers have shown us that seems to be nothing special about the position of the earth. This observation is enshrined in the Copernican cosmological principle: there is nothing special about our vantage point. The laws of physics everywhere else are the same as here, and the universe looks essentially the same from every other point.
102 The Copernican cosmological principle is concerned with sameness in space. The perfect cosmological principle is that the universe looks the same from any point in space and time. The current popularity of the big bang theory weakens the perfect cosmological principle, since it holds that the universe had a definite beginning and has evolved through time, so that things looked different a long time ago.
103 Even given the big bang, however, the perfect cosmological principle holds to the extent that the laws of mathematics and physics have not changed. If they have changed, out attempts to talk about the distant past on the basis of present laws lose credibility.
104 A third cosmological principle is the anthropic (human) cosmological principle, which is just the opposite of the perfect principle. Our existence is an historic fact which (possibly) could have been otherwise. When we look around us (which is equivalent to looking into the past) we see the special history which bore us. If things were different, we would not be here to see them. We are therefore seeing only that part of the universal wilderness which is congenial to our existence.
105 Let us counter the anthropic cosmological principle with the Cantor cosmological principle. As I have described it, the HO models an oscillation between two levels of complexity represented by two transfinite cardinal numbers. The CU is a static structure. We model motion by the HO moving in the CU. The elementary motion in the CU lies between aleph(n) and aleph(n+1). The complexity of this motion is measured by the subscript n. A HO modelling motion of complexity n may be written {aleph(n), aleph(n+1)} and I will call it HO(n).
106 Because Cantor's proof is non-constructive, it simply allows us to distinguish between two possibilities, CT consistent, and CT inconsistent. Each time it is applied it provides us with one bit of information. The fundamental argument of the proof does not change with the complexity n of our position in the CU. All that changes is the input and output of the proof.
107 We may say that CT is invariant with respect to complexity. As Cantor puts it To every transfinite number a there is a next greater proceeding out of it according to a unitary law ... (my emphasis).
108 As I understand it, the inverse of this unitary law is also a unitary law, and so we may say that the the model of knowledge represented by the HO is also invariant with respect to complexity. Insofar as human knowledge is modelled by the HO, it is no different from any other knowledge. From the point of view of the HO, the life and knowledge of a human being is indistinguishable from the life and knowledge of an electron.
109 We should therefore detect the same structures recurring at different levels of complexity. In particular, the Cantor cosmological principle underlies my attempt to take ideas from the simple world of physics and apply them in the complex world of human politics and economy.
110 Finally we arrive at the ergodic cosmological principle, which states that the same information may be encoded in different ways. I noted above that an ergodic source is one whose output in time can be represented by a structure in space. There we were talking about physical space represented by a line, but the term space can be generalised to anything which endures through time. A language may be considered a space in this general sense, and it is common knowledge that the same ideas can be translated into different languages.
111 The ideas behind this essay exist in me, felt but not yet expressed. I am trying to transform them into language. I know that anything I say can be expressed in an infinity of different ways. My task is to get the thing finished in time and in a way that conveys my idea to you. It is very unlikely that I will succeed completely. Every transform of information involves uncertainty, and this fact places a boundary on the universe of each observer. (Back)
Testing
112 Scientific method requires that every physical model must eventually be interpreted in a way that makes it falsifiable. A model may be declared false either if it contains formal inconsistencies, or if it is inconsistent with observation. To test this model, I must make it physical.
113 My theory is that I have a MoG which fits the world I see, so that I can call the universe divine.
114 Why do I call it a MoG? Because I think it meets the specifications for a MoG as they have developed over millennia. As I see it, the basic feature of god is that it is a mystery so far beyond our ken that we can say nothing about it. All that we can say is that it exists, and deny to it those predicates which are inappropriate.
115 The second criterion is that god be consistent and act consistently. This criterion is reflected in the belief current, at least among scientists, that the world is consistent. Every time we falsify a cherished picture the enterprise soldiers on through the confusion toward a new level of consistency whose existence we never doubt.
116 The model implies, I believe, that the observable universe is constrained only by consistency. Does this fit our experience? To sharpen the question a little, I will write down what I see to be the correspondences between the model to date and the reality history has delivered to us.
- It passes as a MoG, as described above;
- It provides us with a non-constructive source of motion (the CS) to play the role of primum movens immobile;
- It establishes a relationship between the discrete and continuous elements of the world, thus allowing motion to coexist with stillness.
- It explains why the arrow of time points toward increasing complexity (entropy) and provides an inverse to this creation in the form of knowledge or abstraction to complete the complexity cycle modelled by the HO. (Back)
How universal is the universe?
117 Since human beings became conscious of themselves and began to record their thoughts in writing we have seen our conception of the size of the universe greatly expand. From Aristotle to Copernicus to Newton to Einstein we have seen the universe grow to an unbounded spacetime 20 billion years across. But has Einstein told us the whole story?
118 At first glance, the CU looks far too big. Even the smallest transfinite number is enough (we think) to count all the particles in the universe. What are we to make of the transfinite hierarchy that lies beyond aleph(0)? How can we cut the model down to size? Do we have to restrict it in some way?
119 The old theologians argued something like this: The universe is explained either by itself or by something else. It is not explained by itself. Therefore it is explained by something else. That something else we call god.
120 The thought dawned on me ever so slowly that the assertion it is not explained by itself may be wrong, a deficiency of contemporary theory rather than an inherent deficiency in the universe. What if the universe could explain itself? Then it is god, and we are parts of god.
121 My theological mentors judged this (correctly) to be heresy in their religious world, and I was duly expelled from their company. It has nevertheless turned out to be a very fruitful line of thought for me. In those days it seemed possible to show that the universe and god are the same by equating them both to an infinite binary string, like the tape of a Turing Machine [see How universal is the universe (1967)]
122 Since then I have come to feel that the proper space for talking about the universe is the CU. How can this abstract model come into existence, that is become the subject of human experience? My initial idea was that the whole universe could be modelled by a sufficiently large and fast universal computer.
123 Suppose operations of this computer manifest as action, a fundamental physical quantity. Quantum mechanics teaches us that there is a smallest quantum of action which is represented by the constant h, discovered by Max Planck in 1900. Assuming a mass M for the universe allows us to estimate the processing rate f of the universal computer from the relationships hf = E = mc squared . A plausible M puts f in the vicinity of 10**100 operations per second.
124 We represent the data and software in such a computer by an infinite binary string. But what of the computer? Specifically, what is the hardware? What does the universe run on?
125 In an ordinary computer a single logical operation involves action by millions of electrons. If each operation of the universal computer involves many micro-operations in the hardware, the search for hardware seems to involve us in an infinite regress, since each operation in the hardware must itself be modelled by the universal computer.
126 So perhaps the software is everything. How can this be? My answer I call logical confinement. The idea is that the processes of the universe are not confined to any hardware, but are carried on within the boundaries of logic itself. What is consistent is observable. What is not consistent ipso facto is not observable. This is essentially the content of Heisenberg's uncertainty principle in the quantum theory.
127 Since CT holds in a logical (consistent) universe, we are provided with a a source and a embarrassing richness of structure in the transfinite cardinal and ordinal numbers.
128 The world, on the other hand, appears finite. Certainly meaningful measurements are finite, and when infinities creep into physical calculations, we are inclined to suspect pathology.
129 If the model represents the transfinite substance of the universe, how do we cut it down to finite observations? The answer I propose lies in the relationship between knower and known. We see the universe as finite because what we see has the same complexity as ourselves. Finity and infinity are relative. This is the idea behind the Cantor Cosmological principle.
130 The theory of relativity tells us that no observer can see or act outside its light cone. Quantum mechanics tells us the the precision of our observation is limited by the quantum of action. (Back)
Locality and Uncertainty
131 I am located in my own present which I take to be the region of spacetime that I can experience. An event in the present is a transition from the future to the past, that is the realisation of a possibility.
132 Future possibilities are infinite and not observable, although they may be predictable from the present. The present is to some degree finite and observable. The past is infinite and not observable, except as it exists in the present. An event, then, is a transition from infinite future to infinite past through the finite present.
133 Now we ask why is the present finite for any observer? The answer is that the observer is part of its own present, and, we will suppose, has the same cardinal number as its own present. The fact is not limited to human beings, but because of its mathematical generality, applies to any particle. I believe that the mathematical notion equivalence which Cantor placed at the foundation of his set theory places a boundary around the present. The position of this boundary is determined by the cardinal number of the present.
134 The idea here is analogous to what computer people call machine infinity. No computer can store a number bigger than its memory. From the point of view of the machine, any number that it cannot store is infinite, and the best it can do is an uncertain approximation. On the other hand I am part of the system, a bigger machine than some, and they therefore look finite to me. (Back)
Relativity
135 The core of relativity theory is a precise definition of what we mean by present in spacetime. Relativity is a local theory, and so contrasts with the theory of Newton. Newton did what he could with the ideas around at the time, but he had to make one big simplification: he assumed instantaneous action at a distance, even though no plausible physical mechanism could achieve this. In the Newtonian picture one can see instantaneously the edges of the universe.
136 Einstein founded his first theory of relativity on the principle that the velocity of light is finite and is the same for all observers in free fall, no matter what the relative velocities of these observers may be. Technically free fall is called inertial motion. In the theory of relativity, local means inertial.
137 What we see depends upon our point of view. The inertial reference frame is the point of view that makes motion in spacetime look simple because it yields fixed points to work from, particularly the local constancy of the velocity of light (c) . In a world of relative motion, we can give no fixed meaning to the symbol at rest. c on the other hand, points to a physical constant. Velocity, meaning distance travelled per unit time, has a definite upper bound.
138 No thing or influence can travel faster than light. This enables us to put limits on the causal relationships that exist between events in spacetime, as illustrated in figure 4.

139 An event in the past can only influence the present if the event is close enough in space for a ray of light to travel from it to the present event. Similarly, the present can only influence an event in the future if it is spatially close enough for as ray of light to travel from the present to the future event. Outside this light cone, there can be no causal relationships between events and the present, so it is labelled mystery.
140 Only massless particles (eg photons) travelling at c can move along the actual light cone, which is a bundle of null geodesics whose spacetime length is zero. This is not obvious from the diagram, which illustrates only one aspect of the whole theory.
141 All points joined by null geodesics are zero spacetime distance apart. This is why we can observe galaxies billions of light years away. The photons entering my eye have experienced no time since they left the star billions of years ago. From the point of view of an observer (who always exists in the present) null geodesics are the boundary of spacetime.
142 This suggests that we can identify photons (particles of light) in the physical world with symbols in the model. Like the symbols of formal mathematical theory, they are eternal, outside spacetime. Photons can be classified into a spectrum parametrized by frequency measured in inverse time. The standard unit of frequency is the Hertz (Hz) , one cycle per second. This spectrum contains as many different photons as there are distinct frequencies.
143 My guess is that this is a countable number, so that we can write n(gamma) = aleph(0) where n(gamma) is the number of categories of photon distinguishable by frequency.
144 Photons move in a massless world, but we massive particles see them from a vantage point where time passes. We express frequency in terms of time, but in the timeless world of the photon, it is a number. Outside spacetime, photons cannot be distinguished by place or time, but they can be distinguished by number. This transformation from information encoded inside spacetime to information encoded on the boundary of spacetime is an application of the ergodic cosmological principle.
145 Both the special and general theories of relativity are classical theories. They model a physical point using the geometric point defined by Euclid: a point is that which has no part (Heath, 153). Their mathematical structure derives from classical analysis which is the branch of mathematics that grew out of the invention of calculus. An important task of analysis is to produce meaningful arguments about infinitesimal quantities, that is quantities arbitrarily close to zero.
146 The resulting mathematical techniques have arbitrarily high precision, since we can get infinitesimally close to where we want to go. This precision led to the idea that we could compute the entire future of the universe if we had adequate knowledge of the present.
147 This idea has turned out to be wrong. The present has a finite size, represented by the box in figure 4. If we want to look into this box we have to turn to quantum mechanics. (Back)
Quantum mechanics
148 The whole structure of the universe, from the point of view of myself or any other particle, depends upon what goes on in my own present.
149 The theory of relativity allows us to look out into the vast spaces of the universe and make sense of them. Quantum mechanics deals with the inner space which is vastly smaller than ourselves.
150 Observation shows that many features of the universe are not defined to arbitrarily high precision. They are fuzzy. The scale of the fuzziness is measured by the quantum of action, h .
151 In relativity the velocity of light represents an outer boundary to our spacetime. We cannot see or be influenced by anything outside our light cone, and the same is true for every other particle in the universe. h represents an inner boundary.
152 We have traced a path from the velocity of light to photons and numbers. To get a good look at the inner boundary we need to talk about computation again. (Back)
Computation
153 A Turing machine, like any other computer, moves through a computation by a series of steps. Each step is a motion which changes the state of the computer. The steps are choreographed by the program, which may be a carefully constructed bit of software designed to achieve a certain result, or simply a set of random numbers. The details of the program do not matter here.
154 Each step is initiated by a clock pulse. We model a clock as a source with an alphabet of two letters emitted alternately. If we assume that the pulses are equiprobable, the entropy of a clock is 1 bit.
155 We are using computation as a model of the universe. Let us assume that the clock of the universe is the simplest HO, that is an HO based on aleph(0) , the first transfinite number. We will name this HO HO(0). Let us further assume that one cycle of HO(0). is equivalent to one quantum of action, h .
156 Now we seem to be back to the hardware/software problem mentioned above. HO(0) cycles between aleph(0) and aleph(1) (fig 3) and so the computer within HO(0) may need to perform something like aleph(1) operations per cycle of HO(0) , each of which would be accompanied by a quantum of action.
157 The way out of this problem seems to be through the new understanding of point introduced by Cantor's set theory and a corresponding boundary to mystery. Euclid's point has no parts. Cantor's point, modelled by a set, may have an infinity of parts, but we abstract from the internal structure of the set and simply consider it as a named entity, analogous to Euclid's point. Whether a given entity behaves as a point or a set is a matter of knowledge, and so a question of interpreting the HO.
158 Just as sets may be elements of sets, HOs may be elements of HOs. Their nested structure reflects the nested structure of the transfinite numbers.
159 In a consistent world there can be no largest set, since CT tells us that any set generates a bigger one. Set theory does admit the existence of a smallest set, however, represented here in the transfinite realm by the smallest transfinite number, so that any aggregate of sets is bounded below.
160 The existence of a least member is a fundamental property of an ordered aggregate and underlies many mathematical proofs, since it gives us a starting point for establishing one to one correspondences. When we are talking about sets, we are talking about the abstract eternal space of mathematics.
161 When we come to talk about the HO, however, we are talking about something like time. Let us imagine that it operates in the opposite way to space, that there is an upper bound to time, but no lower bound. We can make this idea plausible using the ergodic cosmological principle. Since space modelled by the CU has a lower bound but no upper bound, we would expect time to be bounded at one end and unbounded at the other.
162 Now the quantum of action appears to us as neither space nor time but as a mixture of both. In the model it is represented by the substitution of one symbol for another which we call a logical operation. Such a logical operation is a step in a proof or a step in the execution of a computer program.
163 In computing, the size of a step is measured by the complexity of the symbols substituted. The substitution of the symbol 2 for the symbol 1+1 is a simpler step than the substitution of 22 for 11+11. Practical computing also tells us that up to a point a fast computer taking little steps takes the same time to do a particular job as a slow computer taking big steps. There is a tradeoff between speed and wordlength.
164 We have ordered HOs in a spatial way, assigning the name HO(0) to the one with the smallest wordlength aleph(0) . Let us say that HO(0) operates at unbounded frequency, corresponding to the idea that time has no lower bound.
165 Because space has no upper bound, there is no largest HO in this ordering of HOs. If on the other hand we decide to order our HOs by time rather than space, we have no smallest HO but we do have a largest one. From this discussion, we conclude that the size of an HO depends upon whether we look at it from a space point of view or a time point of view or a mixture of both. There is an uncertainty in matching these two points of view whose unit is the quantum of action.
166 This minimum uncertainty gives scale to the universe. It determines the size of fundamental particles, and therefore ultimately of ourselves and everything else.
167 Let us now try to interpret this structure in terms of the law of conservation of energy and the principle of least action, which I feel are the two most general and unifying concepts in physics. (Back)
Conservation of Energy
168 Physics observes that energy is conserved, that is the measure of energy in a closed system does not change with time. Physics recognises two forms of energy, kinetic or energy of motion K and potential or energy of structure or relationship V.
169 An oscillator is a system (like a pendulum) that transfers its energy from K to V and back again. At the top of its swing it is motionless, with minimum K and maximum V. At the bottom if its swing its velocity and K are at a maximum and its V at a minimum. If we average over the cycle, we find that its total energy spends half its time potential and half kinetic.
170 Now let us translate these ideas from the pendulum to the HO (figure 3). We associate kinetic energy with the less complex (lower) side of the oscillator, and potential energy with the more complex (upper) side. The HO moves between structure in space and structure in time. Unlike pendulums (but like quantum oscillators which have similar properties), HOs can be nested as we have discussed above.
171 Physically, action is a product of energy by time. The conservation of energy corresponds to a constant flow of action. We can imagine a set of HOs , H = {HO} with a certain total energy operating between two levels of complexity. Structure in space feeds on structure in time through Cantor's theorem. Structure in time feeds on structure in space through the E-property. The size of H (and therefore the size of the present for H ) is measured by its energy.
172 We can try to imagine the whole ensemble of HOs which constitute the universe. At one end we have the timeless formal aspect which has no maximum size. At the other end we have the spaceless moving aspect which has no limit to the frequency of its motion. Between these two boundaries we have the structure of the CU inhabited by HOs which are a varying mixture of time and space, spacetime as we experience it.
173 The real size and complexity of the CU is beyond our imagination, but it is obvious that we are capable of encapsulating that mysterious splendour in some way because we are sitting here talking about it in what purports to be a meaningful manner. To me it seems a fitting model for the wonders I see when I look out into the universe or into myself. (Back)
The principle of least action
174 There is one more weapon to forge before the arsenal is complete, the principle of least action. Action has a classical history. The principle of least action was first formulated by the French mathematician Maupertuis in 1744. Whenever any action occurs in nature, he said (in French), the quantity of action employed in this change is the least possible. (Yourgrau, 19)
175 Euler established it as an exact dynamical theorem in the same year. The variational method, which seeks understand physical systems by finding the structure corresponding to the least action has proved very fruitful, and may be used to derive the major theories of physics.
176 Here, where we equate the quantum of action and the smallest observable operation of the universal computer, the principle of least action is equivalent to the search for the most efficient program to perform a given action. In Darwinian terms, this most efficient program is the most likely to survive, since it achieves its task with the least resources.
177 The principle of least action or efficient algorithm gives us a means of selecting the observed world out of the immense space of possibility (entropy) represented by the CU.
178 The concept of efficient algorithm, formulated in the general space of the HO, can model an economy as easily as a particle, and so gives us means for expressing the concepts of economic efficiency and economic survival in a competitive system. (Back)
II BANKING LAW
179 We have now a small kit of rather imperfect ideas drawn from the last century of mathematics and physics. It remains to apply them to the law of banking.
Law
180 The old god created the universe because he wanted to. He made it to reflect his own majesty. It is not his child, identical in substance, but a made object, other than and less than god. He gave it natural laws, but these laws are an arbitrary subset of the divine law which constitutes the nature of god.
181 We receive divine law through two channels. First, by the study of natural law, since nature is a reflection of god; and second, by direct revelation from god, for example the laws of the Pentateuch and the supreme law of love given to us by Jesus in the New Testament.
182 A third category of law is that made by human agencies, kings, popes, parliaments and their lawyers: human law.
183 The theological picture presented above blurs these distinctions. By making the universe into god, we have removed the distinction between divine and natural law. We will call this category of law natural, and take it to be the law revealed to us by science.
184 On the other hand, human beings now become part of nature rather than a special creation of god, and so human laws are in a general sense natural laws. They are rather more complex than the laws of physics, but do not differ in principle.
185 Wise human lawmakers act to preserve peace. Less wise ones generally act in their own interests, or at least try to. It is the substance of fairy stories and moral tales that unwise self interest leads to trouble. A wise lawgiver adheres to the perfect cosmological principle and tries to produce laws that apply equally to all persons at all times. (Back)
The rule of law
186
One of the chief currents that can be observed in the history of government is the antinomy between two conflicting forces, power and law. In their pure form, power and law are polar opposites, the former standing for arbitrary might, the latter for a system in which power is checked by institutions or individual rights and channelled in such a way as to conform with a people's values and established patterns of expectation. Neither of these two forces, by itself, can found a stable system of government; one because it is capricious, coercive and unpredictable, the other because, in practice, it can become inflexible and may adapt only with difficulty to changing conditions. Both tend to lead to the buildup of pressures that produce their downfall.
The rule of law (sometimes capitalised as the Rule of Law to distinguish it from legal rules in other contexts) is a legal and constitutional doctrine which reconciles these two antagonistic drives (Walker, 1).
187 In the first section of this paper I have been trying to delineate the principles of the rule of natural law translated into human language by science. An important feature of natural law is that it adheres to the perfect cosmological principle. We assume that similar circumstances will produce a similar outcome regardless of location in spacetime. In modern economic parlance, spacetime is a level playing field .
188 I have taken consistency to be the foundation of natural law, and tried to show (by conceiving the universe as divine) that the laws of nature are not only consistent, but occupy the whole space of consistency as understood by mathematics. An indication of this fullness is the existence of horizons in spacetime beyond which we are faced with mystery. I take these horizons to be observational equivalents of the limits of formal mathematics.
189 I have argued elsewhere [A Theory of Peace/Lecture10/Freedom] that peace in human affairs requires not only that human law be consistent with itself and the world, but that it must allow us to occupy the whole space of consistency . Peace comes when the system is designed for maximum entropy. Trouble comes when we attempt to place an arbitrary limit on human action either locally or globally.
190 In human affairs sheer complexity makes both consistency and the rule of law very difficult to define (Walker, 7-42). It is for this reason that I have turned to the simpler and more formal worlds of mathematics and physics for clues. There I see that law is essentially the boundary between mystery and knowledge. (Back)
Power
191 Power is the hands of individuals can be dangerous because they have a tendency to use it for self interest. The foundation of the rule of law is the distribution of power among the people. This general idea goes under the name democracy, people power.
192 The power of an individual may be manifested in all sorts of ways, but most of them are not measureable, and therefore not the subject of scientific inquiry. What did Hitler have, or Mao, that gave them such influence over human affairs?
193 Modern democracies devolve power to the people through the vote, but any observer of the modern political system can see that the individual right to vote is a tenuous hold on power in the sea of misinformation that passes for political debate.
194 Without meaning to demean the vote, I will here equate power with money. Money is measurable, and so we can measure the power a person wields by the cashflow he controls. (Back)
Money, good and work
195 Money is an abstract representation of good. Good is anything abstract or concrete which a person wants enough to work for. Work is to be understood by analogy to computation as an ordered set of actions designed to possess good.
196 Figure 5 is an economic version of the HO, designed to illustrate the relationship between money and good in the model.
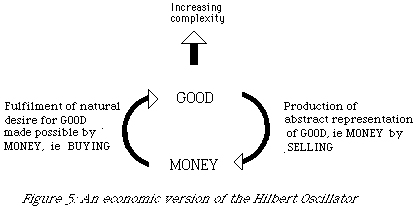
197 Money is considered to be a human invention, but when we look at it closely it has many of the features of action and energy. We have equated power with cashflow. Power is the ability to obtain good.
198 Physically power is measured as energy per unit time. Cashflow is ¢ per unit time, so we can expect money to exhibit features of energy on the buying side of the HO.
199 Money represents good which can be produced by labour. Labour is a flow of action, that is operations per unit time. The simplest method of valuing labour is to pay for time worked, that is action flow is represented by cashflow. Money is thus analogous to action on the selling side of the HO.
200 The principle of least action favours the existence of money, since it greatly increases the ratio of successful transactions to enquiries, so effectively reducing the action necessary to achieve certain sales. (Back)
Banking
201 One of earliest known uses of writing (and hence one of the earliest sciences) was the keeping of accounts, that is the mapping of things and events in the world onto a set of numbers. One may use accounts for inventory control in a barter system, or one may use a pricing system to reduce all items in the inventory to numbers of a common unit, the ¢.
202 Money is an abstract representation of goods, and since it is abstract, we may think of it as knowledge of goods. In a market system, knowledge of prices comes through the market. The movement of goods can be abstractly modelled by the movement of money, so that sets of numbers and arithmetical computations can model the movement of goods. Accounting calculations become much simpler if all value is consolidated in the form of money.
203 It is not a large step from the notion of accounting to that of security. An account is a record of title to some good. Transfer of the good is reflected in a record in the account. A security not only records title to a good, but transfer of security causes the transfer of the good.
204 A bank is a source of money. Originally a banknote was a security entitling the bearer to payment by the bank in gold or some other good on presentation. The banknote issue was secured against the bank's holding of gold.
205 Modern banking has dispensed with gold, and the underlying security of the banking system is now usually the taxing power of the government. (Back)
Tax
206 Tax is a form of protection money. The taxing body contracts in one way or another to deliver peace and possibly welfare in return for payment. The history of taxation, like the history of banking, owes as much to blood and iron as to the rule of law.
207 Now that the last major war is over, however, it may be possible to unite the functions of banking, taxation and the delivery of peace in a global system that delivers the same results for less action and would therefore have a selective advantage over the present system, if ever it were to come into competition with it. (Back)
Consumer sovereignty
208 In the absence of large scale space travel, we can regard the planet earth as a closed system of n natural persons A each with a name Ai (i = 1 ... n). n changes with births and deaths, but that does not affect the story.
209 Each of these people is assumed to know what he wants, either directly or through an appropriate agent. Economically this is known as consumer sovereignty. Advertisers, educators and others may wish to influence these desires, as may people with criminal intent. We presume that these are controlled by an adequate system of accountability and criminal law.
210 Associate with each person a cashflow $i per week. If the value of the system becomes sufficiently obvious, people may provide this information voluntarily. Otherwise we can learn how to do it from existing tax collectors.
211 In the following all statistics are computed on a weekly basis. The global cashflow, referred to human beings GA is thus Sumi $i per week. We can define a corporation C as any entity other than a natural person that has a cashflow and construct a similar measure of global cashflow referred to corporations, CA .
212 Now we can regard the population A as a source whose alphabet is Ai and assign as the probability of each letter the normalised cashflow pi = $i / GA . From this we can compute a figure which we will call the entropy of human cashflow H($i ) = - Sumi pi log pi which will serve as an figure of merit for the cash element of distributive justice.
213 In the present state of the globe, with incomes heavily skewed toward zero, we can expect this figure to be quite low. The next measure is designed to raise it.
214 The entropy of a source increases as the probability of its letters approaches equality. We institute a flat rate tax T equal to a fraction x of individual and corporate cashflow so that the total taxflow T = x( GA + GC ) and (after deducting administrative costs) distribute this money equally to all human individuals A i . It should not be hard to find people to accept their weekly dividend. The system needs some form of identification (eg fingerprint) to prevent too many people being paid twice.
215 This redistribution has two effects. First, it moves income from those with a higher cashflow to those with lower, thus improving the distributive justice measure; and second, it gives every person (and therefore every corporation) current information about the health of the global economy, allowing them to adjust their behaviour accordingly. The tax collection and distribution data can also be processed to yield many other interesting statistics while maintaining anonymity.
216 The divine aspect of money referred to in the title is that in itself it is purely a number, with no respect whatever for persons. We are all equal before the dollar. A major input to the global economic system is solar energy provided free by god, and it seems reasonable that some element of this benefit should shine equally upon us all.
217 This task is to be entrusted to a global bank (the bank of earth, BE ?) whose task, apart from collecting and distributing T is to establish a world currency for its operations, secured against its tax revenue and convertible (at market rates) with all other currencies.
218 In the initial stages of this system, the tax rate x will be very low and its collection and distribution might be subcontracted to existing administrative entities. All existing economic systems may remain undisturbed, except that those governments already administering taxation and social welfare systems may wish to adjust them in the light of the new tax and dividend payments. (Back)
Discussion
219 I believe that the general effect of these measures will be to unite the human population into a single functioning economic entity operating at maximum productivity. This hope is based on the idea of working toward the maximum consistent flow of entropy in the global economic HO.
220 I feel that in time the advantages of this system will lead to the removal of most economic functions from national governments and, with increasing productivity and environmental awareness among consumers, will be the most efficient way to achieve a globally sustainable economy.
221 In time (if the system works) x may grow until reaches a level where everybody can freely purchase many of the services like education and health now delivered to them by the public sector, if at all. The distribution of new consumers around the globe will encourage the growth of business in their vicinity.
222 I feel also that by removing the political stresses caused by uneven distribution it will contribute to peaceful government based on the return of something resembling a tribe (a group of people known to eachother) as the fundamental political entity. The existence of tribes as local administrators will greatly assist the BE in its task.
223 Obviously the next job is to build a computer model of this system and see how it works in competition with the existing system. Unfortunately even rudimentary calculations have been impossible within the time frame of this competition. (Back)
Conclusion
224 The scientific method requires that one accept oneself as a phenomenon in the universe. The old story that we are a defective being as a result of ancestral sin in the garden of Eden will not stand up. The scientific history of our species is far more interesting and encouraging.
225 What is true of an individual in a particular society is partly true of the society as a whole. The world is blighted with ancient religious and political ideas, but the future is uncertain and it is not easy to know what to do about them.
226 Many years ago I was asked to leave a Catholic Monastery for saying too much. At the time I did not know what I was talking about, but I felt strongly that I was onto something and could not retract. Since that time I have been trying to put my feeling into words and this is the latest attempt.
227 Niels Bohr, when asked What is complementary to truth? Answered: Clarity (Pais 1991, p 511). As with non-commuting quantum mechanical variables, one can have one or the other, but it is impossible to get hold of them both at once. I hope the obscurity of this essay suggests the truth of the idea behind it. There is a long way to go yet before I achieve clarity. I thank the organisers of this competition for giving me the incentive to move a little further on the way. (Back)
Further reading
Books
| Abbott, Walter M, The Documents of Vatican II: in a new and definitive translation, with notes and commentaries by Catholic, Protestant and Orthodox authorities, Geoffrey Chapman 1972 Jacket: 'All 16 Documents of Vatican II are here presented in a new and readable translation. Informed comments and appraisals by Catholics and non-Catholics make this book essential reading for anyone, of whatever shade of belief, who is interested in the changing climate of today's world.' Amazon back |
| Aristotle, Metaphysics I-IX, Harvard University Press, William Heinemann 1980 Amazon back |
| Ashby, W Ross, An Introduction to Cybernetics, Methuen 1964 'This book is intended to provide [an introduction to cybernetics]. It starts from common-place and well understood concepts, and proceeds step by step to show how these concepts can be made exact, and how they can be developed until they lead into such subjects as feedback, stability, regulation, ultrastability, information, coding, noise and other cybernetic topics' Amazon back |
| Cantor, Georg, Contributions to the Founding of the Theory of Transfinite Numbers (Translated, with Introduction and Notes by Philip E B Jourdain), Dover 1955 Jacket: 'One of the greatest mathematical classics of all time, this work established a new field of mathematics which was to be of incalculable importance in topology, number theory, analysis, theory of functions, etc, as well as the entire field of modern logic.' Amazon back |
| Chaitin, Gregory J, Information, Randomness & Incompleteness: Papers on Algorithmic Information Theory, World Scientific 1987 Jacket: 'Algorithmic information theory is a branch of computational complexity theory concerned with the size of computer programs rather than with their running time. ... The theory combines features of probability theory, information theory, statistical mechanics and thermodynamics, and recursive function or computability theory. ... [A] major application of algorithmic information theory has been the dramatic new light it throws on Goedel's famous incompleteness theorem and on the limitations of the axiomatic method. ...' Amazon back |
| de Witt, Bryce S and Neill Graham (eds) , The Many-Worlds Interpretation of Quantum Mechanics, Princeton UP 1973 Amazon back |
| Goedel, Kurt, Kurt Goedel: Collected Works Volume 1 Publications 1929-1936, Oxford UP 1986 Jacket: 'Kurt Goedel was the most outstanding logician of the twentieth century, famous for his work on the completeness of logic, the incompleteness of number theory and the consistency of the axiom of choice and the continuum hypotheses. ... The first volume of a comprehensive edition of Goedel's works, this book makes available for the first time in a single source all his publications from 1929 to 1936, including his dissertation. ...' Amazon back |
| Hammond, N G L, The Oxford Classical Dictionary, Clarendon Press 1970-79 Amazon back |
| Heath, Thomas L, Thirteen Books of Euclid's Elements (volume 1, I-II), Dover 1956 'This is the definitive edition of one of the very greatest classics of all time - the full Euclid, not an abridgement. Utilizing the text established by Heiberg, Sir Thomas Heath encompasses almost 2500 years of mathematical and historical study upon Euclid.' Amazon back |
| Hodges, Andrew, Alan Turing: The Enigma, Burnett 1983 Author's note: '... modern papers often employ the usage turing machine. Sinking without a capital letter into the collective mathematical consciousness (as with the abelian group, or the riemannian manifold) is probably the best that scoience can ofer in the way of canonisation.' (530) Amazon back |
| Khinchin, A I, Mathematical Foundations of Information Theory (translated by P A Silvermann and M D Friedman), Dover 1957 Jacket: 'The first comprehensive introduction to information theory, this book places the work begun by Shannon and continued by McMillan, Feinstein and Khinchin on a rigorous mathematical basis. For the first time, mathematicians, statisticians, physicists, cyberneticists and communications engineers are offered a lucid, comprehensive introduction to this rapidly growing field.' Amazon back |
| Kolmogorov, A N , Foundations of the Theory of Probability, Chelsea 1956 back |
| Kramer, Edna E , The Nature and Growth of Modern Mathematics, Princeton UP 1982 Preface: '... traces the development of the most important mathematical concepts from their inception to their present formulation. ... It provides a guide to what is still important in classical mathematics, as well as an introduction to many significant recent developments. (vii) Amazon back |
| McKeon, Richard, The Basic Works of Aristotle, Random 1941 Introduction: 'The influence of Aristotle, in the ... sense of initiating a tradition, has been continuous from his day to the present, for his philosophy contains the first statement, explicit or by opposition, of many of the technical distinctions, definitions, and convictions on which later science and philosophy have been based...' (xi) Amazon back |
| Misner, Charles W, Gravitation, Freeman 1973 Jacket: 'Einstein's description of gravitation as curvature of spacetime led directly to that greatest of all predictions of his theory, that the universe itself is dynamic. Physics still has far to go to come to terms with this amazing fact and what it means for man and his relation to the universe. John Archibald Wheeler. ... this is a book on Einstein's theory of gravity (general relativity).' Amazon back |
| Pais, Abraham, 'Subtle is the Lord...': The Science and Life of Albert Einstein, Oxford UP 1982 Jacket: In this ... major work Abraham Pais, himself an eminent physicist who worked alongside Einstein in the post-war years, traces the development of Einstein's entire ouvre. ... Running through the book is a completely non-scientific biography ... including many letters which appear in English for the first time, as well as other information not published before.' Amazon back |
| Popper, Karl Raimund, The Logic of Scientific Discovery, 1992 Jacket: 'A striking picture of the logical character of scientific discovery is presented here ... Science is presented as ... the attempt to find a coherent theory of the world composed of bold conjectures and disciplines by penetrating criticism.' Amazon back |
| Schilpp, Paul Arthur, Albert Einstein: Philosopher-Scientist, Open Court Publishing Company 1949 Contains Einstein's autobiographical notes in German and English, 25 descriptive and critical essays on the Work of Albert Einstein, Einstein's reply to these essays, and a bibliogrphy of Einstein's writings to May 1951, Amazon back |
| Silk, Joseph, The Big Bang: The Creation and Evolution of the Universe, Freeman 1988 Jacket: 'Written for the non-specialist, The Big Bang describes the greatest contemporary puzzles and achievements in astronomy, cosmology and astrophysics, clearly recounting the history of the universe and examining current controversies from several points of view. The book concludes with a self contained appendix providing the basic mathematical framework for understanding modern cosmology." Amazon back |
| Walker, Geoffrey de Q, The Rule of Law: Foundations of Constitutional Democracy, Melbourne University Press 1988 Jacket: 'The author argues that the survival of any useful rule of law model is currently threatened by distortions in the adjudication process, by perversion of law enforcement (by fabrication of evidence and other means), by the excessive production of new legislation with its degrading effect on long-term legal certainty and on long-standing safeguards, and by legal theories that are hostile to the very concept of rule of law. In practice these trends have produced a great number of legal failures from which we must learn.' Amazon back |
| Yourgrau, Wolfgang, Variational Principles in Dynamics and Quantum Theory, Dover 1979 Amazon back |
Links
| Aquinas Summa: I 3 1: Is God a body? back |
| Aquinas Summa: I 79 4: Is the active intellect something of the soul? back |
Click on an "Amazon" link in the booklist at the foot of the page to buy the book, see more details or search for similar items
Related sites:
Concordat Watch
Revealing Vatican attempts to propagate its religion by international treaty
Copyright: You may copy this material freely provided only that you quote fairly and provide a link (or reference) to your source.